Word Problems: Compound Interest

Related Pages
Interest Word Problems
Investment Word Problems
More Algebra Lessons

Compound Interest Formula

The following diagram gives the Compound Interest Formula. Scroll down the page for more examples and solutions on how to use the compound interest formula.

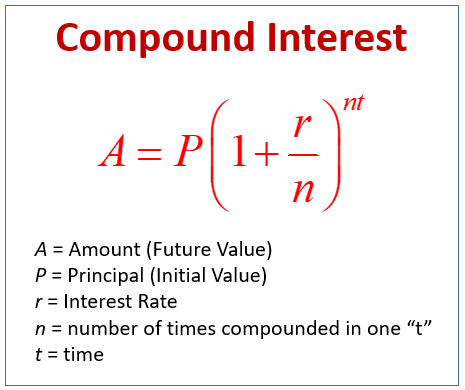
The compound interest formula for compounded interest is:
A = P(1 + r/n)^nt
where A = Future Value
P = Principle (Initial Value)
r = Interest rate
n = number of times compounded in one t
t = time

Examples:

1. Matt is saving for a new car. He invests $5,000 into an account that pays 3% interest a year and is compounded monthly.
   (a) How much will he have after 5 years?
   (b) How long will it take for his investment to double?

2. Matt is planning to buy a car in three years. He want to invest $5,000 now and hopes to have $6,000 to spend on the car when he buys it. What kind of interest rate would he need if his investment is compounded monthly?

What is the compound interest formula for continuously compounded interest?

The following diagram gives the Continuously Compounded Interest Formula. Scroll down the page for more examples and solutions on how to use the Continuously Compounded Interest formula.

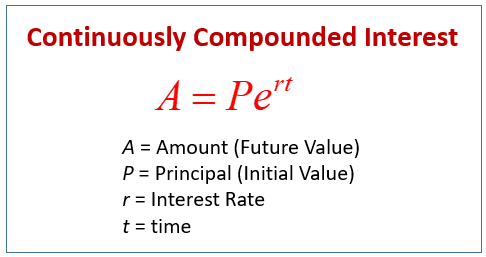
The compound interest formula for continuously compounded interest is
A = Pe^rt
where A = Future Value
P = Principle (Initial Value)
r = Interest rate
t = time

Examples:

1. Lindsey invests $1,000 into an account with 4% per year continuously compounded interest. How much will she have after 10 years? How long will it take for her investment to double?

2. Tony and Matt both invest $5,000 in an account that receives 3% interest annually for 10 years. Tony invests in an account that is compounded monthly. Matt invests in an account that is compounded continuously. Who made the better investment?

How to calculate the effective rate of return?

Example: If $2,500 is invested at 5% compounded monthly, what is the effective rate of return. What is the effective rate of return if this investment is compounded semiannually?

• Show Video Lesson

How to solve word problems involving compound interest and continuously compounded interest?

Examples:

1. Determine the principal P that must be invested at 7% compounded monthly, so that $200,000 will be available for retirement in 15 year.

2. What amount (to the nearest cent) will an account have after 10 years if $175 is invested at 6.5% interest compounded continuously?

3. If $9900 is invested at 14% per annum compounded continuously, how long will it take before the amount is $1,400? Round the answer to two decimal places.

• Show Video Lesson

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.