Illustrative Mathematics Grade 8, Unit 4, Lesson 15: Writing Systems of Equations

Related Pages
Illustrative Math
Grade 8

Lesson 15: Writing Systems of Equations

Let’s write systems of equations from real-world situations.

Illustrative Math Unit 8.4, Lesson 15 (printable worksheets)

Lesson 15 Summary

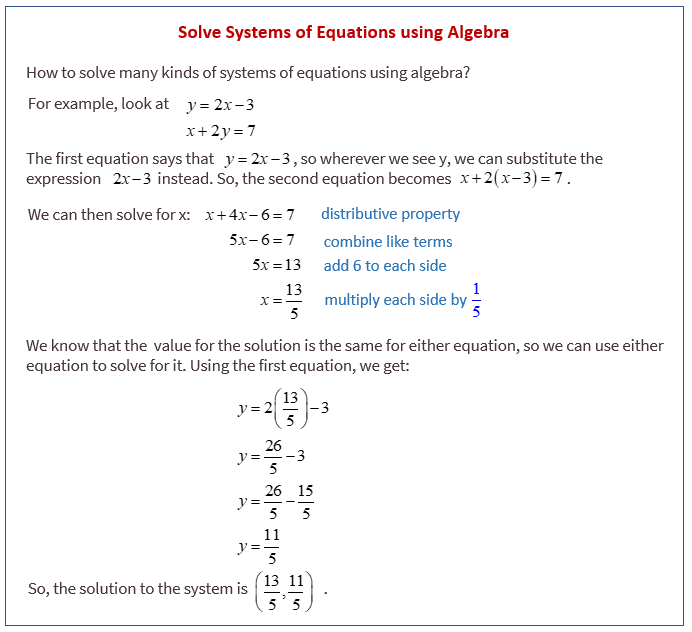
The following diagram show how to solve systems of equations using algebra.

Lesson 15.1 How Many Solutions? Matching

Match each system of equations with the number of solutions the system has.

• See Video for Whole Lesson

Lesson 15.2 Situations and Systems

For each situation:

• Create a system of equations.
• Then, without solving, interpret what the solution to the system would tell you about the situation.

1. Lin’s family is out for a bike ride when her dad stops to take a picture of the scenery. He tells the rest of the family to keep going and that he’ll catch up. Lin’s dad spends 5 minutes taking the photo and then rides at 0.24 miles per minute until he meets up with the rest of the family further along the bike path. Lin and the rest were riding at 0.18 miles per minute.
2. Noah is planning a kayaking trip. Kayak Rental A charges a base fee of $15 plus $4.50 per hour. Kayak Rental B charges a base fee of $12.50 plus $5 per hour.
3. Diego is making a large batch of pastries. The recipe calls for 3 strawberries for every apple. Diego used 52 fruits all together.
4. Flour costs $0.80 per pound and sugar costs $0.50 per pound. An order of flour and sugar weighs 15 pounds and costs $9.00.

Lesson 15.3 Solving Systems Practice

Here is a lot of systems of equations:

1. Without solving, identify 3 systems that you think would be the least difficult for you to solve and 3 systems you think would be the most difficult. Be prepared to explain your reasoning.
2. Choose 4 systems to solve. At least one should be from your “least difficult” list and one should be from your “most difficult” list.

Lesson 15 Practice Problems

1. Kiran and his cousin work during the summer for a landscaping company. Kiran’s cousin has been working for the company longer, so his pay is 30% more than Kiran’s. Last week his cousin worked 27 hours, and Kiran worked 23 hours. Together, they earned $493.85. What is Kiran’s hourly pay? Explain or show your reasoning.
2. Decide which story can be represented by the system of equations y = x + 6 and x + y = 100. Explain your reasoning.
   a. Diego’s teacher writes a test worth 100 points. There are 6 more multiple choice questions than short answer questions.
   b. Lin and her younger cousin measure their heights. They notice that Lin is 6 inches taller, and their heights add up to exactly 100 inches.
3. Clare and Noah play a game in which they earn the same number of points for each goal and lose the same number of points for each penalty. Clare makes 6 goals and 3 penalties, ending the game with 6 points. Noah earns 8 goals and 9 penalties and ends the game with -22 points.
   a. Write a system of equations that describes Clare and Noah’s outcomes. Use to represent the number of points for a goal and to represent the number of points for a penalty.
   b. Solve the system. What does your solution mean?
4. Solve:
5. a. Estimate the coordinates of the point where the two lines meet.
   b. Choose two equations that make up the system represented by the graph.
   c. Solve the system of equations and confirm the accuracy of your estimate.

The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.

Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.